
She realizes that since evaluation is easier than solving, it would be much more convenient to have a different formula, one that takes the Celsius temperature and outputs the Fahrenheit temperature.
In this section, we will consider the reverse nature of functions.Ģ6 = 5 9 ( F − 32 ) 26 ⋅ 9 5 = F − 32 F = 26 ⋅ 9 5 + 32 ≈ 79 26 = 5 9 ( F − 32 ) 26 ⋅ 9 5 = F − 32 F = 26 ⋅ 9 5 + 32 ≈ 79Īfter considering this option for a moment, however, she realizes that solving the equation for each of the temperatures will be awfully tedious.
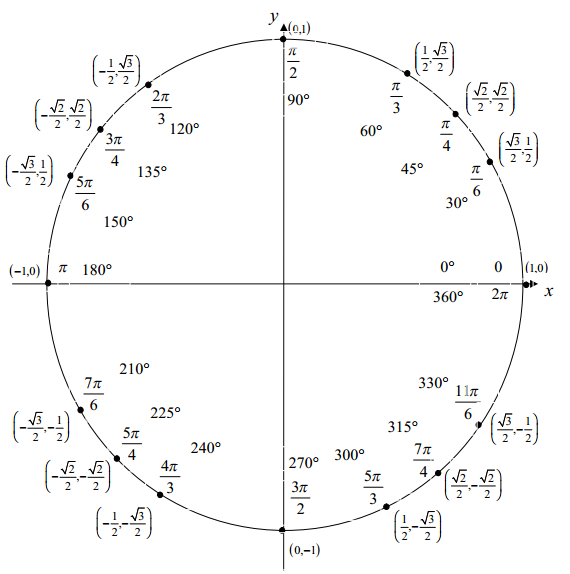
Figure 1 provides a visual representation of this question. If some physical machines can run in two directions, we might ask whether some of the function “machines” we have been studying can also run backwards. As a heater, a heat pump is several times more efficient than conventional electrical resistance heating. Operating in reverse, it pumps heat into the building from the outside, even in cool weather, to provide heating. Operated in one direction, it pumps heat out of a house to provide cooling. Use the graph of a one-to-one function to graph its inverse function on the same axes.Ī reversible heat pump is a climate-control system that is an air conditioner and a heater in a single device.Find or evaluate the inverse of a function.Determine the domain and range of an inverse function, and restrict the domain of a function to make it one-to-one.One must look for the minimum as well as the maximum values of y.Īnswer: A function refers to an expression that shows the relationship between the one variable and another variable.Substitution of different x-values into the expression for y so as to understand what is going on.The range of a function happens to the spread of possible y-values.Question 4: How can one find the range of a function?Īnswer: One can find the range of a function by the following steps:

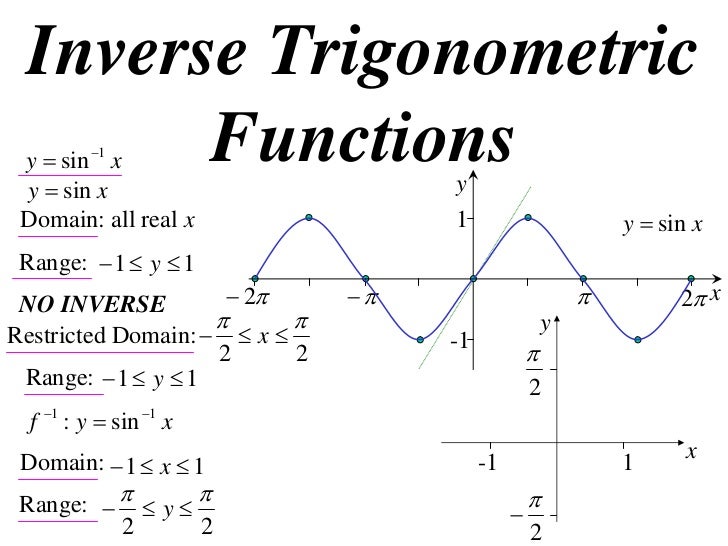
Usually one must avoid 0 on the fraction’s bottom and the negative values which are under the square root sign. Question 3: How can one find the domain of a function?Īnswer: One can determine the domain of each function by looking for independent variables values which one is allowed to use. The range means the set of possible output values whose representation takes place on the y-axis. The domain of a graph involves all the input values which are represented on the x-axis. Question 2: What is meant by domain and range?Īnswer: Domain means the set of possible input values. We also know that for each real number ‘x’, We know that the sine and cosine functions are defined for all real numbers. Domain and Range of Trigonometric Functions


 0 kommentar(er)
0 kommentar(er)
